Motivation

In addition to demonstrating the robustness of the Lagrangian vortex element to capture the growth of instability under severe vorticity stretch, this example shows the flexibility of the method in dealing with complex flow topologies.

One of the (time-consuming) difficulties with predicting (unsteady) external flow problems using traditional grid-based CFD is the issue of determining the extent of the exterior boundaries of the computational domain, such that the choice of the "far-field" boundary conditions will not adversely affect the solution accuracy in the interior. For example, even for the present "simple" problem, the CFD user does not know a priori how far the vortex rings may expand radially subsequent to their collision. As a result, the user may either assign an unnecessarily large domain to get a solution at the first pass or resort to some level of an iterative process to optimize this choice, both of which imply a drop in employee productivity.

In contrast, the far-field boundary conditions are satisfied implicitly in our Lagrangian vortex element simulations, and the solution adaptivity of the method implies that the user need only spend time on discretizing the initial topology of the vorticity field, which is known.

LES of the Collision of Two Vortex Rings

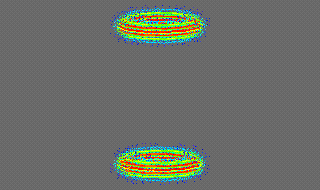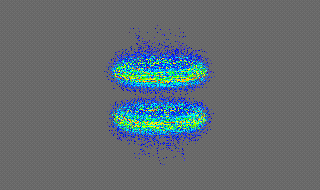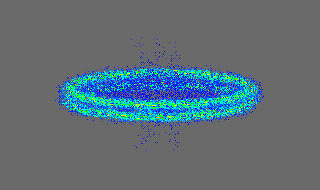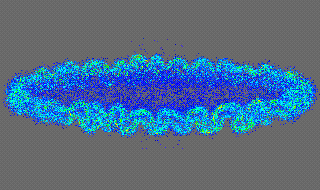
We demonstrate the adaptivity of our method using the LES of the head-on collision of two vortex rings with vortex core to ring radius ratio of 0.275 for both rings. The initial separation between the two rings is equal to 4 ring radii. The vortex core distribution is initially a cubic Gaussian and the rings are perturbed sinusoidally around their azimuth with the most unstable wave-number (=12) and 5% perturbation amplitude. A standard Smagorinsky LES model is used in this case. The rings propagate by their mutually self-induced velocity in free space at infinite Reynolds number. This is an excellent turbulent flow example, which traditional models such as k-e will fail to predict.

The animation at the top of the page depicts the perspective view of the evolution of all vortex particles (the actual computational elements), color coded by the vorticity magnitude (red being the highest and blue being the lowest values). The "sticks" depict the direction of the vorticity vectors.

The animation demonstrates how effortlessly our method deals with the merging of the two rings as they collide, as well as their radial expansion by 3 radii due to a severe vorticity stretch subsequent to the collision process. No special purpose programming was required for this case. Given the template developed for the fat vortex ring problem, the set up of this problem took just a few minutes. This would not be true of traditional CFD methods, because the transient flow topologies for the single vortex and colliding vortex pair problems are fundamentally different!

The stretch during the radial expansion gives rise to substantial increase in enstrophy, and eventually the initial stages of the process of vortex break up and merging into smaller vortex "ringlets" is predicted by our simulation, in agreement with experimental observation. The following is a snapshot of the iso-vorticity surface during the later stages of the simulation.